He is working as an executive vice president - risk management with Yes Bank.

Traditional view on OTC derivatives risk

Until 2008, OTC derivatives focussed on market risk. Counterparty risk was considered secondary. Most counterparties had strong credit rating and the possibility of default was seen as remote. While Basel-II introduced a capital charge for counterparty risk in the trading book and accounting rules introduced in 2006 required counterparty risk to be factored into balance sheet valuations, it continued to be managed at PFE (Potential Future Exposure) level.

Derivatives were valued using the concepts of risk neutral probabilities and no arbitrage. A risk neutral portfolio is expected to earn a risk-free rate and LIBOR rates were the benchmark. The “risk-neutral” or “risk free” price assumed a credit risk free world – where none of the counterparties would default and all contractual cash flows will happen

2008 financial crisis

A cascade of defaults in 2008 (Lehman in particular) exposed the weakness of this traditional view.

Financial institutions and regulators realized that any firm could default and that they had to put much more emphasis in understanding, managing and controlling counterparty risk.

Historically, LIBOR was viewed as the risk free rate, as it was close to AA-rated interbank loans. Post Lehman’s default, the 3-month Fed funds-LIBOR spread widened to 350bps – calling into question the use of LIBOR as benchmark rate. Subsequently, the overnight index swap (OIS) rate has become the “risk-free” rate ¹.

The assumption of no defaults proved to be unrealistic in the post –Lehman world. Financial institutions realized the need to adjust the risk-free price by an amount equivalent to the market price of the counterparty risk embedded in the derivative contract.

Presently, CVA (Credit or Counterparty Value Adjustment) has become very important for financial institutions and they devote substantial resources to calculate CVA in their derivative book. It has been reported that during the 2008/09 financial crisis, two-thirds of the credit related losses that banks suffered were CVA related (paper losses on the balance sheet), as opposed to actual default losses. Once counterparty risk (CVA) is priced, the bank can decide whether to monetize that risk (continue to carry that risk and expect that not too many counterparties will default) or hedge it.

Credit Value Adjustment (CVA)

CVA is an adjustment to the “risk-free” value of a derivative to account for potential counterparty default.

P_{Risky value} = P_{Risk free value} – CVA … (1)

P_(Risky value)=Price of derivative after adjusting for counterparty risk
P_(Risk free value)=Price of derivative without counterparty risk (OIS discounting)
CVA=Couonterparty credit risk adjustment

Historically, CVA was seen as a “credit charge” for pricing and a “reserve” or “provision” for financial reportingpurposes². More recently, CVA is defined as the price of hedging out the counterparty risk, irrespective of default

¹ For example the Fed funds rate in USD – the interest rate at which depository institutions lend balances at the Fed Reserve to other depository institutions overnight. It is considered safer than unsecured deposits (LIBOR loans) because it occurs in the Federal Reserve System under the oversight of the Fed.

² While this doesn’t represent the actual loss for a trade, it’s sufficient in a portfolio context assuming there are many trades across different counterparties 

CVA formula

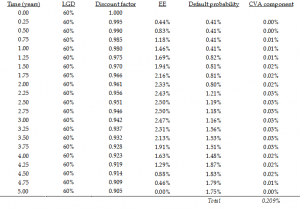
CVA ≈ −LGD ΣB (ti) × EE (ti) × PD (ti − 1, ti)

LGD (Loss given default)= percentage of exposure expected to be lost if the counterparty defaults

B (ti) = risk free discount factor at time ti. Any future losses  must be discounted back to current time

EE (ti) = Expected exposure for the relevant future dates, ti

PD (ti − 1, ti) = Marginal default probability in the interval between (ti-1) and ti

CVA can be expressed either as a standalone value or as a spread (per annum charge).

Example – CVA components for a swap trade

While it can be computed for individual trades, what matters is the CVA of a netting set. This is important because the price of counterparty credit risk needs to mimic what will happen if a counterparty defaults. When the counterparty defaults, the Master Agreements between counterparties will legally put together trades that can be netted off for the liquidation of the portfolio and drive the subsequent payments to and from the defaulted firm. An individual trade should be evaluated only in terms of its contribution to the overall CVA of the netting set.

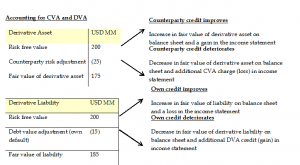
Debt Value Adjustment (DVA) and Bilateral CVA (BCVA)

CVA assumes that the counterparty making the calculation will not default. International accounting standards allow an institution to consider its own default, while valuing its liabilities. Accordingly, the liability component of credit exposure (negative exposure) can be included in the pricing of counterparty risk, as debt value adjustment (DVA)

Bilateral CVA means that an institution will consider its own default, while computing CVA. In the bilateral model, the adjustment to the risk-free value of a derivative is given by

BCVA = CVA + DVA ….(3) where CVA is a cost and DVA is a benefit

Data challenges

Obtaining the necessary market data is a common challenge in CVA computation, especially the default probability and expected exposure components.

CVA computation requires risk neutral probabilities of default. IFRS 13 requires entities to make maximum use of market-observable credit information. CDS spreads may provide a good indication of the market’s perception of counterparty’s creditworthiness. However, many counterparties are “illiquid credits” with no direct market observable measure of creditworthiness. There is a significant subjectivity in obtaining default probabilities for illiquid credits. An even more difficult task is estimating correlations, between market risk factors and credit spreads. These correlations are important in order to be able to model wrong way risk

Exposure quantification is quite difficult over long horizons given the increasing uncertainty about market variables

Regulation and Capital requirements

Basel III rules were introduced in 2009 to strengthen bank capital bases and introduce new requirements on liquidity and leverage. A large portion of the Basel III changes relate to counterparty credit risk and CVA

A capital charge was introduced for CVA volatility (CVA VaR), in addition to the existing charges against counterparty credit risk. This has arisen because a large proportion of the counterparty credit risk related losses in the financial crisis were seen as being mark-to-market based (CVA) rather than due to actual defaults, which were the focus of the Basel II regulations. This had some unintended consequences.

The regulatory focus on CVA seemed to encourage active hedging of counterparty risk so as to obtain capital relief. However, the CDS transactions that were most important for such hedging (single-name and index OTC instruments) introduced their own form of counterparty risk, in particular the wrong way type. The CDS market is even more concentrated than the overall OTC market and has become less, rather than more, liquid in recent years. Since it was the new CVA capital charge that was partially driving the buying of CDS protection that in turn was apparently artificially inflating CDS prices, the methodology for the additional capital charges for counterparty risk has been questioned.